The rectangle is an important two-dimensional shape with many real-world applications. Studying the properties of a rectangle is important to gain an understanding of many of the key concepts used in architecture and design. There are many real-world problems based on calculating the area and perimeter of a rectangle. For instance, in order to determine the cost incurred to fence a rectangular plot, we can use the perimeter of the rectangular plot to evaluate it easily.
Definition of a Rectangle
The word “rectangle” is derived from the Latin word rect (right) and the French word angle (angle). So, a rectangle is a shape that contains four sides intersecting each other at right angles. As the sides of a rectangle are always parallel, it makes the rectangle a type of parallelogram. The opposite sides of a rectangle are always of the same length. From this definition of a rectangle, we can also say that every square is a rectangle because it has four sides meeting each other at right angles. However, not every rectangle is a square since a rectangle’s length, and width does not have to be the same, and that is one of the significant differences between a rectangle and a square. A rectangle is also known as an equiangular quadrilateral.
Properties of Rectangles
A rectangle has many properties that make it a unique geometric figure. Studying these properties enables us to understand rectangles in-depth. Following are some of the most important properties of a rectangle.
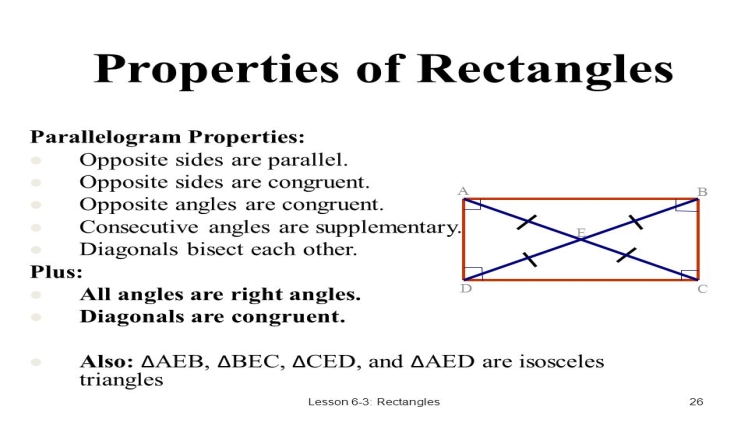
- All the angles of a rectangle are 90 degrees. All the four sides of a rectangle always intersect at a right angle; thus, the four angles created when a rectangle is formed are always 90 degrees.
- Opposite sides of a rectangle are equal and Parallel; one of the most distinctive properties of a rectangle is that its sides are of equal length. The angle between the sides is always 90 degrees, and opposite sides are always parallel.
- Diagonals of a rectangle bisect each other. A diagonal is a line that is formed by joining the opposite corners of the rectangle. In a rectangle, the two diagonals are created when we join opposite corners. The diagonal of the rectangle divides the rectangle into two right triangles.
- The sum of all the internal angles of a triangle is always 360 degrees. Also, the sum of opposite angles of a triangle is always 180 degrees.
Perimeter Of A Rectangle
Perimeter is the sum of the length of all the sides of a rectangle. Since opposite sides of a rectangle are always equal, the length of only two sides is enough to find the rectangle’s perimeter.
Perimeter is the distance around a geometric shape. To calculate the perimeter of a rectangle, we need the dimension of the sides of the rectangle. The opposite sides of the rectangle are always equal, so the length of two adjacent sides is required to calculate the perimeter of the rectangle. Once the length of any two adjacent sides is known, the rectangle’s perimeter can be calculated by using formula 2(Length + Breadth).
Area of a Rectangle
The area of rectangle is the space covered inside the sides of a rectangle. The concept of the area of a rectangle is important in many real-life calculations. For example, we can apply the formula of the area of a rectangle to find the space occupied by various rectangular objects like paintings, notebooks, laptop screens, LCDs, etc.
To calculate the area of a rectangle, we can use this formula:
The area of a rectangle is equal to its length times its width = Length X Breadth ( L x B )
Follow the steps mentioned below to find the area of a rectangle.
Step 1: Take the provided dimensions of length and breadth.
Step 2: Multiply the value of length and breadth.
Step 3: Proffer the answer in square units.